Conditional probability (条件概率) 是A-Level数学课程考点概率章节中很多同学容易出错的知识点。由于条件的变化多端，导致概率计算时常常需要考虑事件发生的前提，那么今天我们就展开说说”条件概率”的那些事儿。

　　首先我们来看两个问题D设随机试验E为”抛一颗公平骰子，观察出现的点数”，求点数为1的概你设随机试验F为”抛一颗公平器观察出现的点数”已知点数为奇数，求点数为1的概率。

　　这两问当中，只存在一个变化，就是”点数为奇数”这个条件，D问当中的sample space (样本空间) 为(1,2,3,4,5,6。那么在“点数为奇数”这个前提下，@问的sample space的缩减为(1,3,5}。所以，D问中的概率为一;@问中的概率为一。

　　通过以上简单的例子，我们可以总结出，条件概率的计算原理就是建立在样本空间的变化之上的 (通常为样本数量的减少)

　　Conditional probability的表达形式

　　我们用P(AB)来表示:在B事件发生的前提下，A事件发生的概率。那么我们前面所提到的2问就可以表示为:P (x=1/x is odd)在实际做题当中要注意标志性词汇:“given that”。它后面的即为条件 (前提)。

　　真题演练

　　To gain a place at a science college, students first have to pass a written test and then a practical testEach student is allowed a maximum of two attempts at the written test.

　　A student is only alloweca second attempt if they fail the first attempt, No student is allowed more than one attempt at thepractical test. If a student fails both attempts at the written test, then they cannot attempt the practicatest.The probability that a student will pass the written test at the first attempt is 0.8. If a student fails thefirst attempt at the written test.

　　the probability that they will pass at the second attempt is 0.6. Theprobability that a student will pass the practical test is always 0.3.

　　(a) Draw a tree diagram to represent this information,showing the probabilities on the branches.

　　简要翻译:本题提到两类考试:笔试和实践。笔试有两次机会，通过笔试即可参加实践考试，笔试两次失败则不能参加实践考试。笔试首考通过概率为0.8，二考通过概率为0.6，实践考试通过概率为0.3。用树形图表达。

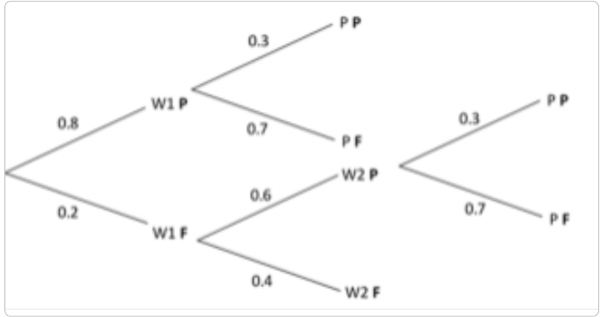
　　树形图中W代表笔试，P代表实践考试，1&2代表笔试次数，黑体的P&F分别代表通过和失败。(b)Find the probability that a randomly chosen student will succeed in gaining a place at the college.